13. Volume
e1a. Volume using Thin Cylinders - Example
The area below the function \(y=3\sin x\) between \(x=0\) and \(x=\pi\) is rotated about the \(y\)-axis producing a solid of revolution shaped like a jello mold. Find the volume.
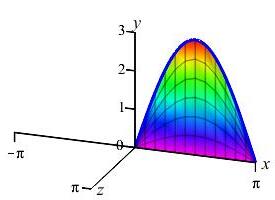
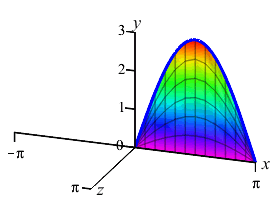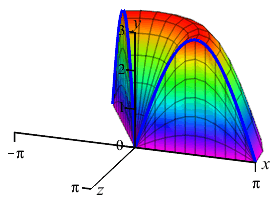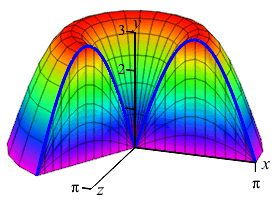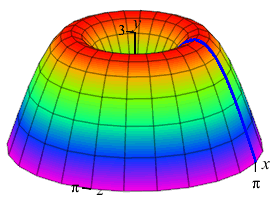
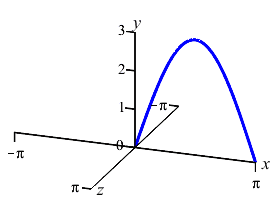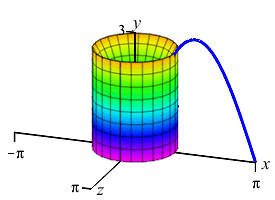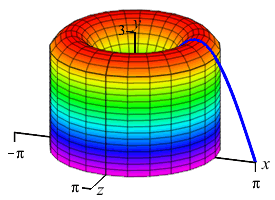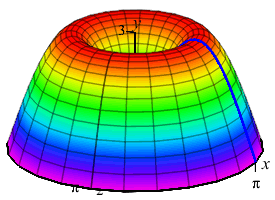
We do not want to compute the volume as a \(y\) integral because that would require integrals of \(\arcsin\)'s. So we do an \(x\)-integral. The rectangles are vertical and rotate into thin cylinders. The last plot shows the thin cylinders accumulating to form the solid.
The radius is \(x\) and the height is \(3\sin x\). So the volume is \[ V=\int_0^\pi 2\pi x\cdot3\sin x\,dx \]

To do the integral, we must use integration by parts with \[\begin{array}{ll} u=6\pi x & dv=\sin x\,dx \\ du=6\pi\,dx \quad & v=-\cos x \end{array}\] So the volume is: \[\begin{aligned} V&=\left[-6\pi x\cos x+\int 6\pi\cos x\,dx\right]_0^\pi \\ &=\left[-6\pi x\cos x+6\pi\sin x\rule{0pt}{10pt}\right]_0^\pi =-6\pi\pi\cos\pi=6\pi^2 \end{aligned}\]